Our New Solar Cell Paradigm

    In the Madhukar group, we are investigating a new solar cell architecture that bypasses the limitations of charge carrier extraction and transport by utilizing nonradiative dipole-dipole coupling for direct transfer of energy (NRET) from the excitons created in the solar absorber to high charge carrier mobility transport channels.

    In this new solar cell architecture, shown schematically in figure 1 below, the excitons are created by light absorption in nanocrystal quantum dot absorbers and their energy transferred nonradiatively to an adjacent high carrier mobility transport medium via direct dipole-dipole interaction between the NCQD and the adjacent transport channel states. The tranfer rate is given by the Forster expression

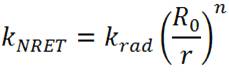
in which kNRET is the nonradiative energy transfer rate, krad is the radiative decay rate, R0 the Forster Critical Radius, r is the separation between dipoles, and n depends on the dimensionality of the acceptor. This mechanism is distinct from those employed in solar cells of any type (excitonic or otherwise) so far. Semiconductor quantum wells and quantum or nanowires are known to provide high carrier mobility channels [7]. Such channels, quantum wells or wires, are provided by the technology of the planar or spatially-patterned epitaxial [8-10] quantum wells and wires (Fig.1a) or chemically templated [11, 12] wires (nano or quantum) formed perpendicular to the substrate (Fig.1b). Common to all such architectures, the fundamental physical process to be examined and established then is the controlled nonradiative transfer of excitation energy from the NCQD absorbers to nearby quantum wells and wires. We emphasize that this process is separate from the break-up of the exciton at a heterojunction and attendant carrier (electron or hole) transfer to a transport channel as is the case with current excitonic solar cells, or radiative energy transfer to the channel.

Figure 1. Schematic showing a new solar cell architecture utilizing nonradiative coupling between dipoles for direct transfer of energy from the excitons created in the light absorber to (a) quantum well (b) nanowire high mobility charge carrier transport channels.

To assess the viability of hte new approach, we have measured the nonradiaitve energy transfer rate between nanocrystal quantum dots and adjacent quantum well, as described below.


Energy transfer in hybrid nanocrystal quantum dot / adjacent quantum well systems


    For efficient nonradiative transfer of energy, appropriate matching of the absorption and emission spectra of the donor and acceptor species and their separation is a critical consideration [13]. For our studies, PbS NCQDs are employed as the absorbers (donors) and an appropriately designed adjacent InGaAs quantum well buried in GaAs matrix provides the high mobility charge transport channel for accepting the resonantly transferred exciton energy in the form of electron and hole. To shed light on the time scale and efficiency of excitation transfer from the PbS NCQD into the InGaAs near surface quantum well, we have examined the time resolved behavior of the luminescence decay from the NCQDs adsorbed on quantum well containing substrates and compared it to the behavior on control substrates without the buried quantum well.

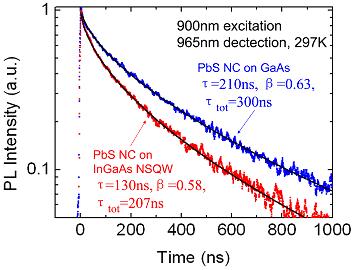
Fig. 2. Time resolved PL of PbS NCs on passivated GaAs (blue) and on passivated NSQW (Red). Excited at 900nm (below GaAs bandgap) and detected at 965nm (PbS PL peak). TRPL curves are fitted using stretched exponential function.

    Figure 2 shows the room temperature time decay behavior of the luminescence peak of the NCQDs at 965nm. Note the considerably fast decay time of ~207ns in the presence of the quantum well as compared to the ~300ns decay time on the GaAs control substrate. The reduction of the NCQD PL decay time from ~300ns to ~207ns is the manifestation of the opening of an excitation transfer channel provided by the one-dimensionally confined states of the quantum well (Fig.2). From the difference between these two measured decay times, we calculate the nonradiative transfer rate to be ~ 1/(690ns), ~1.4 times faster than their radiative decay rate ~ 1/(960ns) measured for the NCQDs dispersed on a glass substrate. This means for a nanocrystal of 100% quantum yield, the efficiency of the nonradiative transfer from the NCQD to the quantum well is ~60% for the 8.2 nm center-to-center separation of the PbS NCQDs adn the InGaAs QW in these experiments. The transfer efficiency is expected to be further improved by reducing the distance between the NCQDs and the NSQW.

For a full description of the findings, see our paper:
Siyuan Lu, Anupam Madhukar, "Nonradiative Resonant Excitation Transfer from Nanocrystal Quantum Dots to Adjacent Quantum Channels", Nano Letters, 7, 3443-3451 (2007). [CLICK HERE]

Charge Carrier Generation and Photocurrent in Silicon Nanowires by NRET from Adjacent Quantum Dots

To demonstrate the feasibility of using energy transfer to generate current in high mobility transport channels, we have measured the time resolved photocurrent in silicon nanowires - PbS QD hybrid structures as represented in figure 3a below. Photocurrent measurements for devices with and without QDs are shown in figure 3b. The electron-hole pairs generated in the silicon nanowires due to direct photon absorption in the nanowires will be swept away extremely rapidly by a modest electric field across the wires. By contrast, the NRET time being several hundred nanoseconds [14], its contribution to the photocurrent will occur on a similarly long time scale, thus separating the contribution to the total measured photocurrent into its two components, those electrons and holes generated owing to NRET, as shown in the blue curve in figure 3c, and those generated due to direct absorption in nanowires, as shown in the black curve in figure 3c. Integrating the curves in figure 3c shows that the contribution to current from NRET can be three times greater than that from direct absorption in the nanowires.

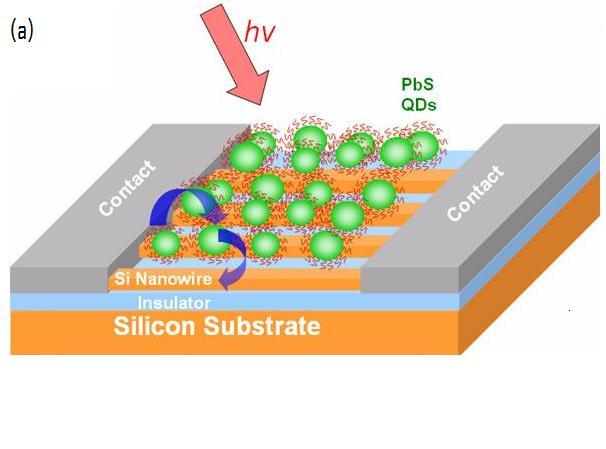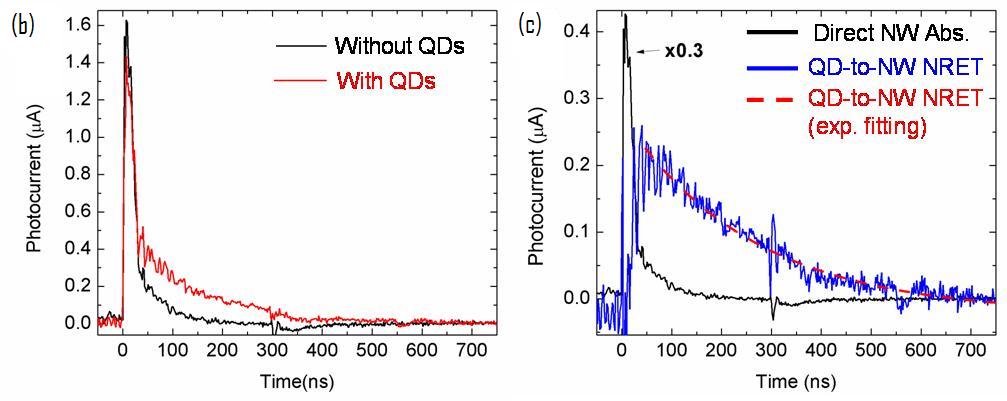
Figure 3: (a) Schematic of silicon nanowire arrays with PbS QDs used in time resolved photocurrent measurements. (b) Measured photocurrent for nanowire array wth (red) and without PbS QDs (black) . (c) Components of photocurrent due to direct absorption in nanawires (black) and photocurrent from PbS QD to nanowire energy tranfer (blue).

For a full description, see our paper:

S. Lu, Z. Lingley, T. Asano, D. Harris, T. Barwicz, S. Guha, and A. Madhukar, " Photocurrent Induced by Nonradiative Energy Transfer from Nanocrystal Quantum Dots to Adjacent Silicon Nanowire Conducting Channels: Towards a New Solar Cell Paradigm" Nano Lett., 9 , 4548-4552 (2009) [CLICK HERE]

Enhanced Interdot Energy Transfer by Reduction of QD-QD Separation by Cation-Ligand Exchange

Efficient collection of current requires that energy from light absorbed in QDs many layers away from the quantum well or wire surface can be efficiently transfered dot-to-dot to eventually be transfered from a QD into the well/wire. To enhance interdot energy transfer we have developed a method of ligand exchange that allows for control and reduction of the separation between adjacent PbS QD when the QDs are in densely packed solids. Our ligand exchange is unique in that is utilizes lead cation - carboxylate ligands as exchange units rather than plain ligands as is most commonly used, and the value of this apporach is that it does not significantly degrade the quantum efficiency of the PbS quantum dots. Exchanging the relatively long oleate ligands on as-grown PbS QDs with shorter dodecatoate and octanoate ligands lead to a decrease in QD-QD spacing and an increase in the interdot energy transfer rate, kNRET. In fact, we demonstrated that the energy transfer rate depends on the inverse sixth power (n =6 for QD to QD energy transfer) of interdot sepration, r, as expected from the Forster expression above.

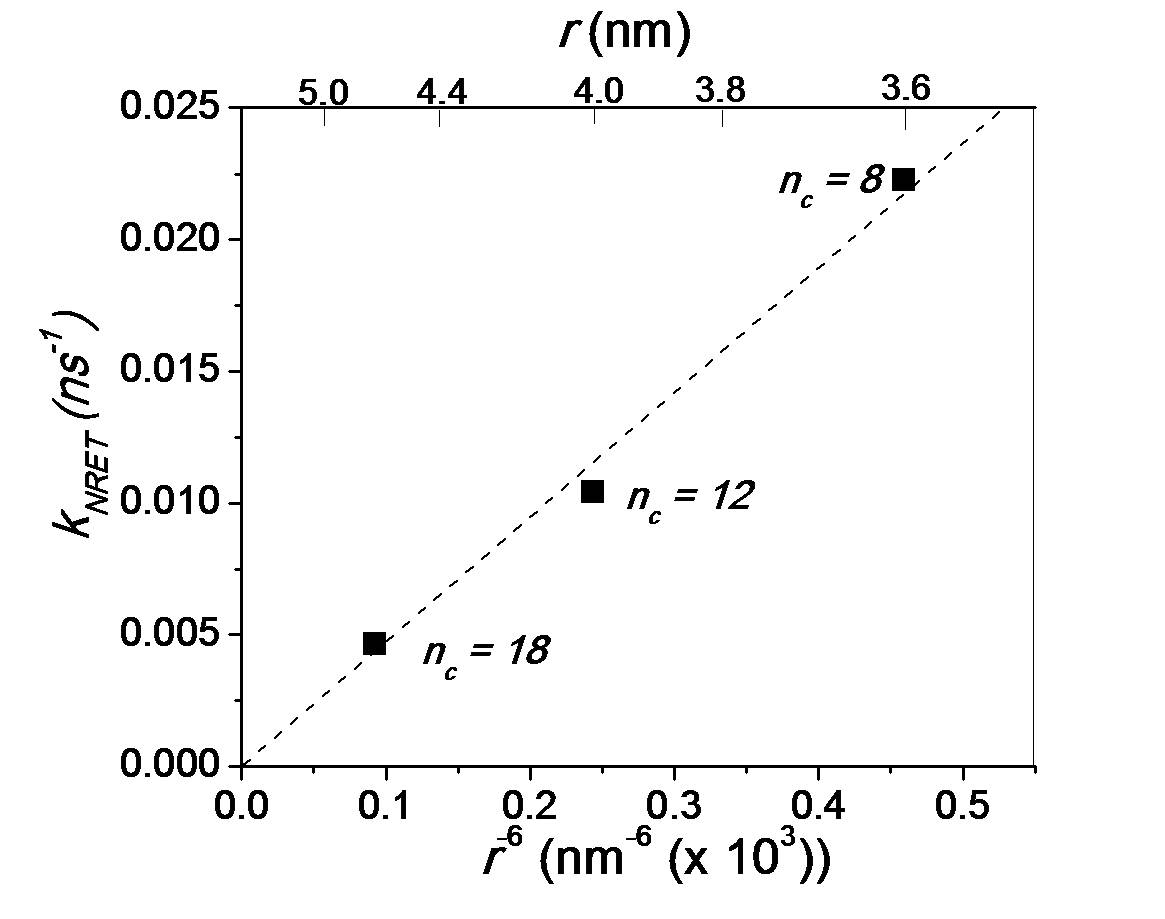
Figure 4: Interdot energy transfer rate, kNRET, as a function of QD-QD separation, r, to the inverse sixth power. nc refers to the number of carbon atoms in the carboxylate ligand attached to the QD surface.

For a full description, see our paper:

Z. Lingley, S. Lu. and A. Madhukar, "A High Quantum Efficiency Preserving Approach to Ligand Exchange on Lead Sulfide Quantum Dots and Interdot Resonant Energy Transfer" Nano Lett., 11, 2887-2891 (2011) [CLICK HERE]

References:
[1] Shockley, W.; and Queisser, H. J.; Jour. App. Phys. 1963, 32, 510-519.
[2] King, R. R.; Law, D. C.; Edmondson, K. M.; Fetzer, C. M.; Kinsey, G. S.; Yoon, H.; Sherif, R. A.; Karam, N. H.; Appl. Phys. Lett., 2007, 90, 183516.
[3] Oregan, B.; Gratzel, M.; Nature, 1991, 353, 737-740.
[4] Hoppe, H.; Sariciftci, N. S.; J. Mater. Res. 2004, 19, 1924-1945.
[5] Kim, J. Y. ; Lee, K. ; Coates, N. E. ; Moses, D. ; Nguyen, T. Q. ; Dante, M. ; Heeger, A. J. ; Science 2007, 317, 222-225.
[6] Nozik, A. J. Physica E, 2002, 14, 115; Gur, I.; Fromer, N. A.; Alivisatos, A. P.; J. Phys. Chem. B 2006, 110, 25543-25546.
[7] Weisbuch, C. Fundamental properties of III-V semiconductor two-dimensional quantized structures: The basis for optical and electronic device applications, Ch.1 in Semiconductors and Semimetals: Application of Multiquantum Wells, Selective Doping, and Superlattices, Ed. R. Dingle, Academic Press, New York, (1987); Interfaces, Quantum Wells, and Superlattices, Eds, C. R. Leavens and R. Taylor Plenum, New York, (1988).
[8] Madhukar, A.; Thin Solid Films, 1993, 231, 8-42.
[9] Konkar, A.; Madhukar, A.; Chen, P.; Appl. Phys. Lett., 1998, 72, 220-222.
[10] Kiravittaya, S.; Heidemeyer, H.; Schmidt, O. G.; Physica E, 2004, 23, 253-259.
[11] Westwater, J.; Gosain, D. P.; Tomiya, S.; Usui, S.; Ruda, H. J.; Vac. Sci. Technol. B, 1997, 15, 554-557.
[12] Dick, K. A.; Deppert, K.; Karlsson, L. S.; Wallenberg, L. R.; Samuelson, L.; Seifert, W.; Advanced Functional Materials, 2005, 15, 1603-1610; Zhong, Z. H.; Qian, F.; Wang, D. L.; Lieber, C. M.; Nano Lett., 2003, 3, 343-346; Kim, Y.; Joyce, H. J.; Gao, Q.; Tan, H. H.; Jagadish, C.; Paladugu, M.; Zou, J.; Suvorova, A.; Nano Lett., 2006, 6,599 - 604.
[13] Förster, Th.; Annu. Rev. Phys., 1948, 2, 55-75; Förster, Th.; Discuss. Faraday Soc., 1959, 27, 7-17.
[14] Lu, S.; Madhukar, A.; Nano Lett. 2007, 7, 3443-3451.